 login/create account
login/create account
coloring
Seagull problem ★★★
Author(s): Seymour
 vertex graph with no independent set of size
vertex graph with no independent set of size 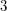 has a complete graph on
has a complete graph on 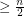 vertices as a minor.
vertices as a minor. Keywords: coloring; complete graph; minor
Coloring squares of hypercubes ★★
Author(s): Wan
If  is a simple graph, we let
is a simple graph, we let 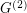 denote the simple graph with vertex set
denote the simple graph with vertex set 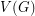 and two vertices adjacent if they are distance
and two vertices adjacent if they are distance 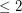 in
in  .
.
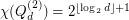 .
. Unfriendly partitions ★★★
If  is a graph, we say that a partition of
is a graph, we say that a partition of 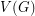 is unfriendly if every vertex has at least as many neighbors in the other classes as in its own.
is unfriendly if every vertex has at least as many neighbors in the other classes as in its own.
Keywords: coloring; infinite graph; partition
5-coloring graphs with small crossing & clique numbers ★★
For a graph  , we let
, we let 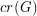 denote the crossing number of
denote the crossing number of  , and we let
, and we let 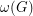 denote the size of the largest complete subgraph of
denote the size of the largest complete subgraph of  .
.
 with
with 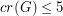 and
and 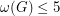 have a 5-coloring?
have a 5-coloring? Keywords: coloring; crossing number; planar graph
Coloring the Odd Distance Graph ★★★
Author(s): Rosenfeld
The Odd Distance Graph, denoted  , is the graph with vertex set
, is the graph with vertex set 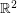 and two points adjacent if the distance between them is an odd integer.
and two points adjacent if the distance between them is an odd integer.
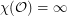 ?
? Keywords: coloring; geometric graph; odd distance
Coloring and immersion ★★★
Author(s): Abu-Khzam; Langston
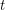 , every (loopless) graph
, every (loopless) graph  with
with 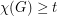 immerses
immerses 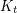 .
. Keywords: coloring; complete graph; immersion
Bounding the chromatic number of graphs with no odd hole ★★★
Author(s): Gyarfas
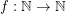 so that
so that 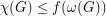 for every graph
for every graph  with no odd hole.
with no odd hole. Keywords: chi-bounded; coloring; induced subgraph; odd hole; perfect graph
5-local-tensions ★★
Author(s): DeVos
 (probably
(probably 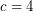 suffices) so that every embedded (loopless) graph with edge-width
suffices) so that every embedded (loopless) graph with edge-width 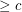 has a 5-local-tension.
has a 5-local-tension. Alon-Saks-Seymour Conjecture ★★★
Author(s): Alon; Saks; Seymour
 is a simple graph which can be written as an union of
is a simple graph which can be written as an union of  edge-disjoint complete bipartite graphs, then
edge-disjoint complete bipartite graphs, then 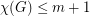 .
. Keywords: coloring; complete bipartite graph; eigenvalues; interlacing
Bounded colorings for planar graphs ★★
Author(s): Alon; Ding; Oporowski; Vertigan
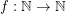 so that every planar graph of maximum degree
so that every planar graph of maximum degree  has a partition of its vertex set into at most three sets
has a partition of its vertex set into at most three sets 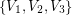 so that for
so that for 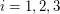 , every component of the graph induced by
, every component of the graph induced by 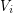 has size at most
has size at most 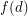 ?
? Keywords: coloring; partition; planar graph

 Drupal
Drupal CSI of Charles University
CSI of Charles University