 login/create account
login/create account
minor
Number of Cliques in Minor-Closed Classes ★★
Author(s): Wood
Question Is there a constant  such that every
such that every  -vertex
-vertex 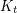 -minor-free graph has at most
-minor-free graph has at most 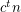 cliques?
cliques?
 such that every
such that every  -vertex
-vertex 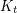 -minor-free graph has at most
-minor-free graph has at most 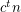 cliques?
cliques? Seagull problem ★★★
Author(s): Seymour
Conjecture Every  vertex graph with no independent set of size
vertex graph with no independent set of size 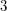 has a complete graph on
has a complete graph on 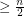 vertices as a minor.
vertices as a minor.
 vertex graph with no independent set of size
vertex graph with no independent set of size 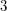 has a complete graph on
has a complete graph on 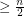 vertices as a minor.
vertices as a minor. Keywords: coloring; complete graph; minor
Seymour's self-minor conjecture ★★★
Author(s): Seymour
Conjecture Every infinite graph is a proper minor of itself.
Keywords: infinite graph; minor
Consecutive non-orientable embedding obstructions ★★★
Author(s):
Conjecture Is there a graph  that is a minor-minimal obstruction for two non-orientable surfaces?
that is a minor-minimal obstruction for two non-orientable surfaces?
 that is a minor-minimal obstruction for two non-orientable surfaces?
that is a minor-minimal obstruction for two non-orientable surfaces? Highly connected graphs with no K_n minor ★★★
Author(s): Thomas
Problem Is it true for all 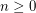 , that every sufficiently large
, that every sufficiently large  -connected graph without a
-connected graph without a  minor has a set of
minor has a set of 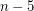 vertices whose deletion results in a planar graph?
vertices whose deletion results in a planar graph?
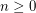 , that every sufficiently large
, that every sufficiently large  -connected graph without a
-connected graph without a  minor has a set of
minor has a set of 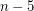 vertices whose deletion results in a planar graph?
vertices whose deletion results in a planar graph? Keywords: connectivity; minor

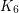
 Drupal
Drupal CSI of Charles University
CSI of Charles University