 login/create account
login/create account
Edge coloring
Packing T-joins ★★
Author(s): DeVos
 (probably
(probably 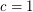 suffices) so that every graft with minimum
suffices) so that every graft with minimum 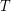 -cut size at least
-cut size at least  contains a
contains a 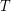 -join packing of size at least
-join packing of size at least 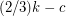 .
. Acyclic edge-colouring ★★
Author(s): Fiamcik
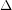 has a proper
has a proper 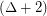 -edge-colouring so that every cycle contains edges of at least three distinct colours.
-edge-colouring so that every cycle contains edges of at least three distinct colours. Keywords: edge-coloring
A generalization of Vizing's Theorem? ★★
Author(s): Rosenfeld
 be a simple
be a simple  -uniform hypergraph, and assume that every set of
-uniform hypergraph, and assume that every set of 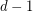 points is contained in at most
points is contained in at most  edges. Then there exists an
edges. Then there exists an 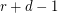 -edge-coloring so that any two edges which share
-edge-coloring so that any two edges which share 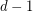 vertices have distinct colors.
vertices have distinct colors. Keywords: edge-coloring; hypergraph; Vizing
List colorings of edge-critical graphs ★★
Author(s): Mohar
 is a
is a 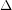 -edge-critical graph. Suppose that for each edge
-edge-critical graph. Suppose that for each edge  of
of  , there is a list
, there is a list 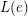 of
of 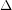 colors. Then
colors. Then  is
is 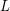 -edge-colorable unless all lists are equal to each other.
-edge-colorable unless all lists are equal to each other. Keywords: edge-coloring; list coloring
Universal Steiner triple systems ★★
Author(s): Grannell; Griggs; Knor; Skoviera
Keywords: cubic graph; Steiner triple system
Edge list coloring conjecture ★★★
Author(s):
 be a loopless multigraph. Then the edge chromatic number of
be a loopless multigraph. Then the edge chromatic number of  equals the list edge chromatic number of
equals the list edge chromatic number of  .
. Keywords:
Seymour's r-graph conjecture ★★★
Author(s): Seymour
An  -graph is an
-graph is an  -regular graph
-regular graph  with the property that
with the property that 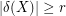 for every
for every 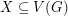 with odd size.
with odd size.
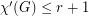 for every
for every  -graph
-graph  .
. Keywords: edge-coloring; r-graph
Goldberg's conjecture ★★★
Author(s): Goldberg
The overfull parameter is defined as follows: ![\[ w(G) = \max_{H \subseteq G} \left\lceil \frac{ |E(H)| }{ \lfloor \tfrac{1}{2} |V(H)| \rfloor} \right\rceil. \]](/files/tex/d2391343543ce03d861e6eb2f4985d52e309525d.png)
 satisfies
satisfies 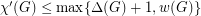 .
. Keywords: edge-coloring; multigraph
Strong edge colouring conjecture ★★
A strong edge-colouring of a graph  is a edge-colouring in which every colour class is an induced matching; that is, any two vertices belonging to distinct edges with the same colour are not adjacent. The strong chromatic index
is a edge-colouring in which every colour class is an induced matching; that is, any two vertices belonging to distinct edges with the same colour are not adjacent. The strong chromatic index 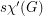 is the minimum number of colours in a strong edge-colouring of
is the minimum number of colours in a strong edge-colouring of  .
.
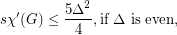
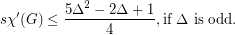
Keywords:

 Drupal
Drupal CSI of Charles University
CSI of Charles University