 login/create account
login/create account
S(S(f)) = S(f) for reloids
See Algebraic General Topology, especially Connectedness of funcoids and reloids for definitions of used concepts.
Bibliography
*Victor Porton. Algebraic General Topology
* indicates original appearance(s) of problem.

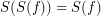 for every endo-
for every endo-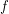 ?
?  Drupal
Drupal CSI of Charles University
CSI of Charles University
Mistake fixed
There were a big mistake in http://www.mathematics21.org/binaries/connectedness.pdf where are defined some of the concepts used by this open problem. I have rewritten the article, not it should be OK.
--
Victor Porton - http://www.mathematics21.org