 login/create account
login/create account
Conjecture For all positive integers 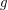 and
and  , there exists an integer
, there exists an integer  such that every graph of average degree at least
such that every graph of average degree at least  contains a subgraph of average degree at least
contains a subgraph of average degree at least  and girth greater than
and girth greater than 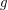 .
.
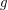 and
and  , there exists an integer
, there exists an integer  such that every graph of average degree at least
such that every graph of average degree at least  contains a subgraph of average degree at least
contains a subgraph of average degree at least  and girth greater than
and girth greater than 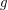 .
. This conjecture is true for regular graphs as observed by Alon (see [KO]). The case 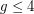 was proved in [KO].
was proved in [KO].
Bibliography
[KO] D. Kühn and D. Osthus, Every graph of sufficiently large average degree contains a C4-free subgraph of large average degree, Combinatorica, 24 (2004), 155-162.
*[T] C. Thomassen, Girth in graphs, J. Combin. Theory B 35 (1983), 129–141.
* indicates original appearance(s) of problem.

 Drupal
Drupal CSI of Charles University
CSI of Charles University