 login/create account
login/create account
Recent Activity
A funcoid related to directed topological spaces ★★
Author(s): Porton
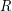 be the complete funcoid corresponding to the usual topology on extended real line
be the complete funcoid corresponding to the usual topology on extended real line ![$ [-\infty,+\infty] = \mathbb{R}\cup\{-\infty,+\infty\} $](/files/tex/3252019c60a83f00ff396d823dbff8040639f409.png) . Let
. Let 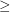 be the order on this set. Then
be the order on this set. Then 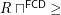 is a complete funcoid.
is a complete funcoid. 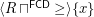 is the infinitely small right neighborhood filter of point
is the infinitely small right neighborhood filter of point ![$ x\in[-\infty,+\infty] $](/files/tex/4e57a21194d8d5a659e259a111ed13a9c23b52a1.png) .
. If proved true, the conjecture then can be generalized to a wider class of posets.
Keywords:
Infinite distributivity of meet over join for a principal funcoid ★★
Author(s): Porton
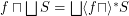 for principal funcoid
for principal funcoid 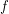 and a set
and a set  of funcoids of appropriate sources and destinations.
of funcoids of appropriate sources and destinations. Keywords: distributivity; principal funcoid
Weak saturation of the cube in the clique ★
Determine 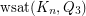 .
.
Keywords: bootstrap percolation; hypercube; Weak saturation
Convex Equipartitions with Extreme Perimeter ★★
Author(s): Nandakumar
To divide a given 2D convex region C into a specified number n of convex pieces all of equal area (perimeters could be different) such that the total perimeter of pieces is (1) maximized (2) minimized.
Remark: It appears maximizing the total perimeter is the easier problem.
Keywords: convex equipartition
Turán Problem for $10$-Cycles in the Hypercube ★★
Author(s): Erdos
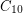 in the hypercube.
in the hypercube. Keywords: cycles; extremal combinatorics; hypercube
Extremal $4$-Neighbour Bootstrap Percolation in the Hypercube ★★
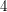 -neighbour bootstrap process in the hypercube.
-neighbour bootstrap process in the hypercube. Keywords: bootstrap percolation; extremal combinatorics; hypercube; percolation
Saturation in the Hypercube ★★
Author(s): Morrison; Noel; Scott
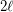 in the
in the  -dimensional hypercube?
-dimensional hypercube? Keywords: cycles; hypercube; minimum saturation; saturation
Cycles in Graphs of Large Chromatic Number ★★
Author(s): Brewster; McGuinness; Moore; Noel
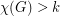 , then
, then  contains at least
contains at least 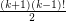 cycles of length
cycles of length 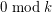 .
. Keywords: chromatic number; cycles
The Double Cap Conjecture ★★
Author(s): Kalai
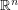 containing no pair of orthogonal vectors is attained by two open caps of geodesic radius
containing no pair of orthogonal vectors is attained by two open caps of geodesic radius 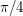 around the north and south poles.
around the north and south poles. Keywords: combinatorial geometry; independent set; orthogonality; projective plane; sphere
Circular flow numbers of $r$-graphs ★★
Author(s): Steffen
A nowhere-zero  -flow
-flow 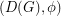 on
on  is an orientation
is an orientation 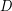 of
of  together with a function
together with a function 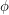 from the edge set of
from the edge set of  into the real numbers such that
into the real numbers such that 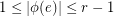 , for all
, for all  , and
, and  .
.
A 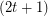 -regular graph
-regular graph  is a
is a 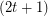 -graph if
-graph if 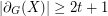 for every
for every 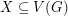 with
with 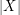 odd.
odd.
 be an integer. If
be an integer. If  is a
is a 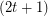 -graph, then
-graph, then 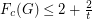 .
. Keywords: flow conjectures; nowhere-zero flows
Circular flow number of regular class 1 graphs ★★
Author(s): Steffen
A nowhere-zero  -flow
-flow 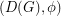 on
on  is an orientation
is an orientation 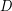 of
of  together with a function
together with a function 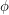 from the edge set of
from the edge set of  into the real numbers such that
into the real numbers such that 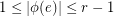 , for all
, for all  , and
, and  . The circular flow number of
. The circular flow number of  is inf
is inf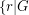 has a nowhere-zero
has a nowhere-zero  -flow
-flow 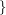 , and it is denoted by
, and it is denoted by 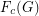 .
.
A graph with maximum vertex degree  is a class 1 graph if its edge chromatic number is
is a class 1 graph if its edge chromatic number is  .
.
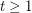 be an integer and
be an integer and  a
a 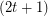 -regular graph. If
-regular graph. If  is a class 1 graph, then
is a class 1 graph, then 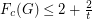 .
. Chromatic number of associahedron ★★
Author(s): Fabila-Monroy; Flores-Penaloza; Huemer; Hurtado; Urrutia; Wood
Are there infinite number of Mersenne Primes? ★★★★
Author(s):
![\[ M_n = 2^p - 1 \]](/files/tex/eb18a56e5c2e8b1be6ac733d217c0c1f1a47a94e.png) that is prime.
that is prime.
Are there infinite number of Mersenne Primes?
Keywords: Mersenne number; Mersenne prime
Are all Mersenne Numbers with prime exponent square-free? ★★★
Author(s):
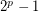 Square free?
Square free? Keywords: Mersenne number
What are hyperfuncoids isomorphic to? ★★
Author(s): Porton
Let 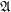 be an indexed family of sets.
be an indexed family of sets.
Products are 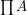 for
for 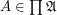 .
.
Hyperfuncoids are filters 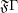 on the lattice
on the lattice  of all finite unions of products.
of all finite unions of products.
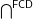 a bijection from hyperfuncoids
a bijection from hyperfuncoids 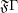 to:
to:- \item prestaroids on
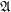 ; \item staroids on
; \item staroids on 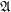 ; \item completary staroids on
; \item completary staroids on 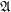 ?
? If yes, is 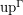 defining the inverse bijection? If not, characterize the image of the function
defining the inverse bijection? If not, characterize the image of the function 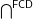 defined on
defined on 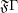 .
.
Consider also the variant of this problem with the set  replaced with the set
replaced with the set 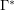 of complements of elements of the set
of complements of elements of the set  .
.
Keywords: hyperfuncoids; multidimensional
Another conjecture about reloids and funcoids ★★
Author(s): Porton
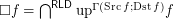 for reloid
for reloid 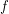 .
.  for every funcoid
for every funcoid 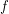 .
. Note: it is known that 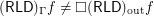 (see below mentioned online article).
(see below mentioned online article).
Keywords:
Inequality for square summable complex series ★★
Author(s): Retkes
 the following inequality holds
the following inequality holds 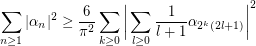
Keywords: Inequality
One-way functions exist ★★★★
Author(s):
Keywords: one way function
Chromatic Number of Common Graphs ★★
Author(s): Hatami; Hladký; Kráľ; Norine; Razborov
Keywords: common graph
Erdős–Straus conjecture ★★
For all 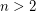 , there exist positive integers
, there exist positive integers  ,
, 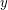 ,
,  such that
such that 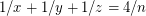 .
.
Keywords: Egyptian fraction

 Drupal
Drupal CSI of Charles University
CSI of Charles University