 login/create account
login/create account
Recent Activity
Vertex Cover Integrality Gap ★★
Author(s): Atserias
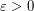 there is
there is 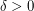 such that, for every large
such that, for every large  , there are
, there are  -vertex graphs
-vertex graphs  and
and  such that
such that 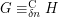 and
and  .
. Keywords: counting quantifiers; FMT12-LesHouches
Big Line or Big Clique in Planar Point Sets ★★
Let  be a set of points in the plane. Two points
be a set of points in the plane. Two points  and
and  in
in  are visible with respect to
are visible with respect to  if the line segment between
if the line segment between  and
and  contains no other point in
contains no other point in  .
.
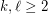 there is an integer
there is an integer  such that every set of at least
such that every set of at least  points in the plane contains at least
points in the plane contains at least 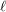 collinear points or
collinear points or  pairwise visible points.
pairwise visible points. Keywords: Discrete Geometry; Geometric Ramsey Theory
Mixing Circular Colourings ★
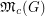 always rational?
always rational? Keywords: discrete homotopy; graph colourings; mixing
The Borodin-Kostochka Conjecture ★★
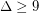 has chromatic number at most
has chromatic number at most  .
. Keywords:
Chromatic number of random lifts of complete graphs ★★
Author(s): Amit
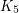 concentrated on a single value?
concentrated on a single value? Keywords: random lifts, coloring
3 is a primitive root modulo primes of the form 16 q^4 + 1, where q>3 is prime ★★
Author(s):
Keywords:
Circular choosability of planar graphs ★
Author(s): Mohar
Let 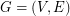 be a graph. If
be a graph. If 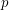 and
and 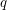 are two integers, a
are two integers, a 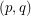 -colouring of
-colouring of  is a function
is a function  from
from 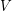 to
to 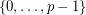 such that
such that 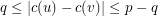 for each edge
for each edge 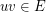 . Given a list assignment
. Given a list assignment 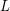 of
of  , i.e.~a mapping that assigns to every vertex
, i.e.~a mapping that assigns to every vertex  a set of non-negative integers, an
a set of non-negative integers, an 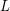 -colouring of
-colouring of  is a mapping
is a mapping 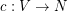 such that
such that 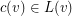 for every
for every 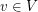 . A list assignment
. A list assignment 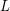 is a
is a 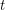 -
-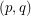 -list-assignment if
-list-assignment if 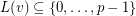 and
and 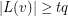 for each vertex
for each vertex 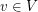 . Given such a list assignment
. Given such a list assignment 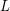 , the graph G is
, the graph G is 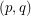 -
-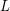 -colourable if there exists a
-colourable if there exists a 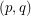 -
-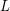 -colouring
-colouring  , i.e.
, i.e.  is both a
is both a 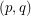 -colouring and an
-colouring and an 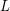 -colouring. For any real number
-colouring. For any real number 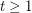 , the graph
, the graph  is
is 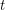 -
-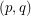 -choosable if it is
-choosable if it is 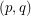 -
-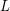 -colourable for every
-colourable for every 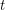 -
-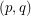 -list-assignment
-list-assignment 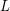 . Last,
. Last,  is circularly
is circularly 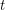 -choosable if it is
-choosable if it is 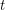 -
-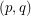 -choosable for any
-choosable for any 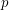 ,
, 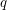 . The circular choosability (or circular list chromatic number or circular choice number) of G is
. The circular choosability (or circular list chromatic number or circular choice number) of G is 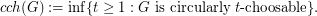
Keywords: choosability; circular colouring; planar graphs
A conjecture about direct product of funcoids ★★
Author(s): Porton
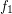 and
and 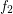 are monovalued, entirely defined funcoids with
are monovalued, entirely defined funcoids with 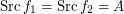 . Then there exists a pointfree funcoid
. Then there exists a pointfree funcoid 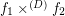 such that (for every filter
such that (for every filter  on
on 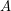 )
) 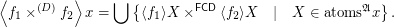 (The join operation is taken on the lattice of filters with reversed order.)
(The join operation is taken on the lattice of filters with reversed order.) A positive solution of this problem may open a way to prove that some funcoids-related categories are cartesian closed.
Keywords: category theory; general topology
MacEachen Conjecture ★
Author(s): McEachen
Keywords: primality; prime distribution
Criterion for boundedness of power series ★
Author(s): Rüdinger
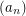 so that the power series
so that the power series 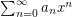 is bounded for all
is bounded for all 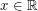 .
. Keywords: boundedness; power series; real analysis
Length of surreal product ★
Author(s): Gonshor
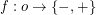 , where
, where  is some ordinal. This
is some ordinal. This  is the length of given sign expansion and also the birthday of the corresponding surreal number. Let us denote this length of
is the length of given sign expansion and also the birthday of the corresponding surreal number. Let us denote this length of  as
as 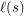 .
.
It is easy to prove that
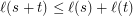
What about
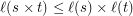
?
Keywords: surreal numbers
Durer's Conjecture ★★★
Convex uniform 5-polytopes ★★
Author(s):
Keywords:
MSO alternation hierarchy over pictures ★★
Author(s): Grandjean
Keywords: FMT12-LesHouches; MSO, alternation hierarchy; picture languages
Blatter-Specker Theorem for ternary relations ★★
Author(s): Makowsky
Let 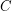 be a class of finite relational structures. We denote by
be a class of finite relational structures. We denote by  the number of structures in
the number of structures in 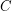 over the labeled set
over the labeled set 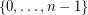 . For any class
. For any class 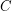 definable in monadic second-order logic with unary and binary relation symbols, Specker and Blatter showed that, for every
definable in monadic second-order logic with unary and binary relation symbols, Specker and Blatter showed that, for every  , the function
, the function  is ultimately periodic modulo
is ultimately periodic modulo  .
.
Keywords: Blatter-Specker Theorem; FMT00-Luminy
Monadic second-order logic with cardinality predicates ★★
Author(s): Courcelle
The problem concerns the extension of Monadic Second Order Logic (over a binary relation representing the edge relation) with the following atomic formulas:
- \item
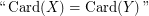 \item
\item 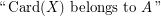
where 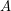 is a fixed recursive set of integers.
is a fixed recursive set of integers.
Let us fix  and a closed formula
and a closed formula 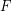 in this language.
in this language.
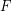 for a graph
for a graph  of tree-width at most
of tree-width at most  can be tested in polynomial time in the size of
can be tested in polynomial time in the size of  ?
? Keywords: bounded tree width; cardinality predicates; FMT03-Bedlewo; MSO
Order-invariant queries ★★
Author(s): Segoufin
- \item Does
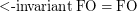 hold over graphs of bounded tree-width? \item Is
hold over graphs of bounded tree-width? \item Is 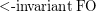 included in
included in 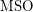 over graphs? \item Does
over graphs? \item Does 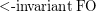 have a 0-1 law? \item Are properties of
have a 0-1 law? \item Are properties of 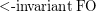 Hanf-local? \item Is there a logic (with an effective syntax) that captures
Hanf-local? \item Is there a logic (with an effective syntax) that captures 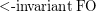 ?
? Keywords: Effective syntax; FMT12-LesHouches; Locality; MSO; Order invariance
Fixed-point logic with counting ★★
Author(s): Blass
- \item Given a graph, does it have a perfect matching, i.e., a set
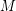 of edges such that every vertex is incident to exactly one edge from
of edges such that every vertex is incident to exactly one edge from 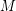 ? \item Given a square matrix over a finite field (regarded as a structure in the natural way, as described in [BGS02]), what is its determinant?
? \item Given a square matrix over a finite field (regarded as a structure in the natural way, as described in [BGS02]), what is its determinant? Keywords: Capturing PTime; counting quantifiers; Fixed-point logic; FMT03-Bedlewo
Birch & Swinnerton-Dyer conjecture ★★★★
Author(s):
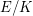 be an elliptic curve over a number field
be an elliptic curve over a number field 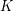 . Then the order of the zeros of its
. Then the order of the zeros of its 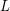 -function,
-function, 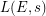 , at
, at 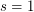 is the Mordell-Weil rank of
is the Mordell-Weil rank of 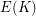 .
. Keywords:

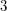 is a
is a 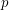 for all primes
for all primes 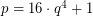 , where
, where 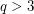 is prime.
is prime.  and
and  are
are  Drupal
Drupal CSI of Charles University
CSI of Charles University